Hello and welcome to my ongoing video course about Multidimensional Integration, already consisting of 11 videos. This series will guide you through the key concepts, step by step, to help you understand the intricacies of multidimensional integrals. Along with the videos, you’ll find helpful text explanations. You can test your knowledge using the quizzes and refer to the PDF versions of the lessons whenever needed. If you have any questions, feel free to ask in the community forum. Let’s get started!
Part 1 - Lebesgue Measure and Lebesgue Integral
Let’s start the video series by stating the definition of the important Lebesgue measure. To understand the whole construction, you have to watch my Measure Theory course. However, even if you didn’t watch it, you should grasp the essential properties this measure has. The conclusion in the end is that we have a much more powerful integral on the real number line. This one generalizes the Riemann integral, you might know from Real Analysis.
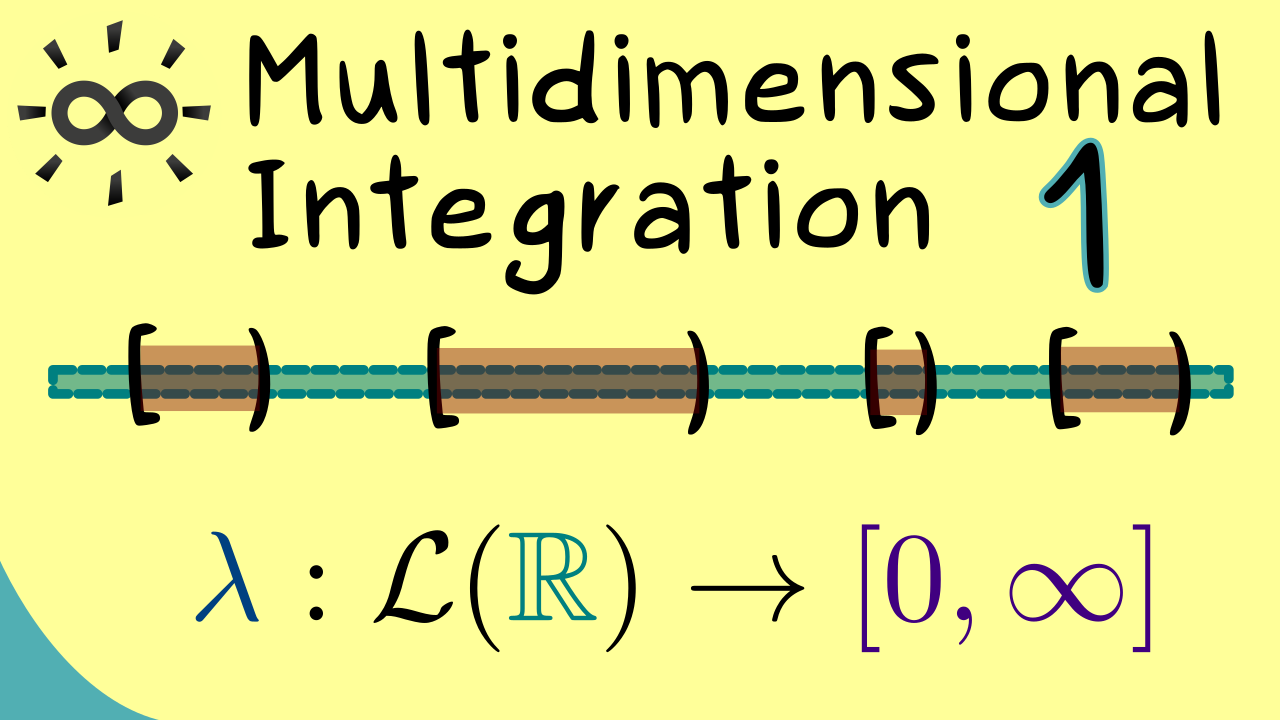
Part 2 - The n-dimensional Lebesgue Measure
In the second part, the power of the general Lebesgue integral comes really through. It’s no problem at all to generalize everything quickly to higher dimensions. This is something where the Riemann integral really struggles with, but with the Lebesgue integral, everything is simple and fast.
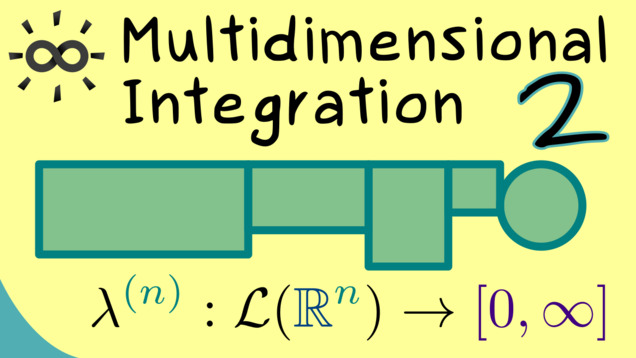
Part 3 - Fubini’s Theorem
You might already know that higher-dimensional integrals can be solved by transforming them into one-dimensional integral and solving them. This procedure is known as Fubini’s theorem or Fubini-Tonelli theorem. It works under mild assumptions for the functions such that one can say that it is really a universal tool to solve integrals. In this video, we will discuss these assumptions and the correct formulation.
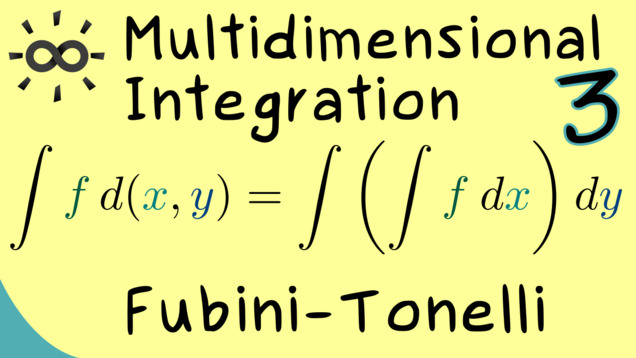
Part 4 - Fubini’s Theorem in Action
We present the usage of Fubini’s theorem by considering a simple two-dimensional example.
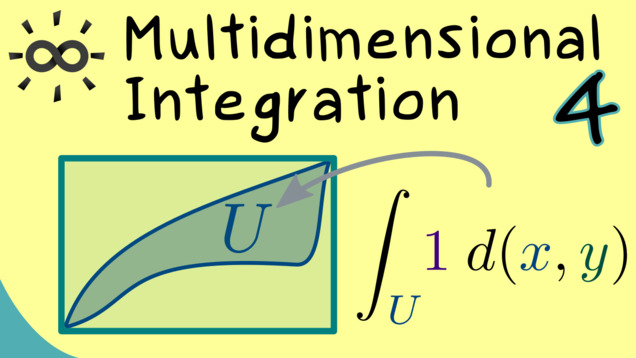
Part 5 - Change of Variables Formula
As an important integration technique for solving one-dimensional integrals, we know the so-called substitution rule. It turns out that one can generalize that to $n$-dimensional integrals as well and there it is known as the change of variables formula. The key ingredient there is the Jacobian determinant of a chosen diffeomorphism, often called $ \Phi $.
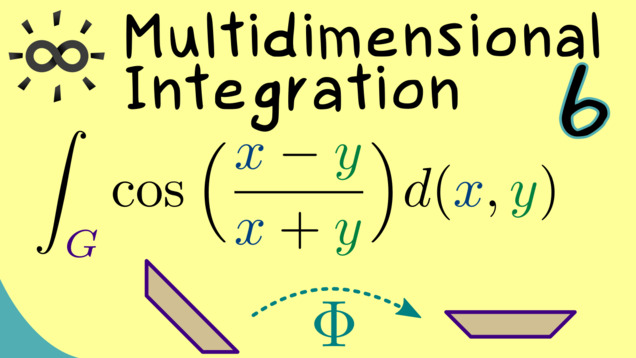
Part 6 - Example for Change of Variables
Let’s look at an example calculation for the change of variables formula and also for Fubini’s theorem.
Part 7 - Surface Integral
Until now, we have only considered volume integrals in $\mathbb{R}^n$ as a generalization of the ordinary one-dimensional integral in $\mathbb{R}$. However, there is another concept of integration where you want to intgerate along a curve or over a surface. This requires a different approach for the integral because such a surface does not have be flat like $\mathbb{R}^n$. We will define this so-called surface integral by looking at tangent vectors, normal vectors, and the Gramian determinant.
Part 8 - Example of Surface Integral
We can immediately apply the definition of the surface integral to the 2-dimensional sphere in $\mathbb{R}^3$. You might already know the spherical coordinates one can use for that. In particular, we can take them to calculate the Gramian determinant.
Part 9 - Integration with Polar Coordinates
While working in $\mathbb{R}^n$, it can definitely happen that a problem carries some symmetries where spherical coordinates would make the calculation easier. In the general case, this is known as integrating in polar coordinates. It turns out that the surface integral over the sphere helps a lot in formulating such an $n$-dimensional integration formula.
Part 10 - Divergence Theorem
A classical result in vector analysis is Gauss’s Integral Theorem also know as the Divergence Theorem because it’s connects the divergence of a vector field to a surface integral. We discuss some typical notations but don’t show the proof of this theorem since it follows from a general theorem discussed in the Manifolds series.
Other videos related to this topic:
Part 11 - Green’s Identities
It turns out that the Divergence Theorem from the last video directly gives us a formula that we could call integration by parts in higher dimensions. There we see how to integrate a product of functions by shifting a derivative from one function to another one. As in the one-dimensional case, a boundary term also occurs. This is will be used to derive two statements known as Green’s first identity and Green’s seconds identity.
Summary of the course Multidimensional Integration
-
You can download the whole PDF here and the whole dark PDF.
-
You can download the whole printable PDF here.
- Ask your questions in the community forum about Multidimensional Integration.
Ad-free version available:
Click to watch the series on Vimeo.