Here, you find my whole video series about Measure Theory in the correct order and I also help you with some text around the videos. If you have any questions, you can use the comments below and ask anything. As a motivation about the whole topic, the Lebegue integral is often used and, there, the question arises why we actually need a new integral notion when we already in calculus how to integrate. For this motivation, I’ve created a video you find here. However, without further ado let’s start with this series here:
Part 1 - Sigma Algebras
If you want to learn Measure Theory and understand the general Lebesgue Integral, you first need to know what a Sigma-algebra is:
Content of the video:
00:00 Introduction
00:58 Measuring lengths
03:00 Example power set
03:51 Definition sigma-algebra
10:20 Example for sigma-algebras
Now you know that a Sigma-algebra is just a special collection of subsets. Of course, if I give you just any collection of subsets, you are able to check if this one satisfies the three rules above. If this is not the case, you can add new sets into the collection such that the result is a sigma-algebra. In the next video you see how this works in a theoretical way (generated Sigma-algebra).
Part 2 - Borel Sigma Algebras
One other collection of subsets is given in a topology: open sets! With these, we can generate a very important Sigma-algebra: the Borel Sigma-algebra.
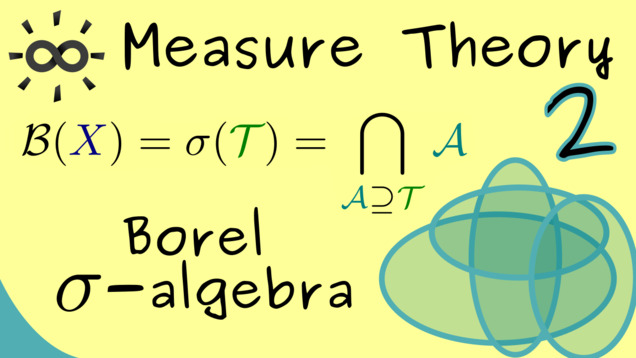
Content of the video:
00:00 Recap sigma algebra
00:50 Intersections of sigma algebra
02:18 Generated sigma algebra
04:38 Example
08:30 Definition Borel sigma algebra
Part 3 - What is a measure?
You might have already noted that the whole point of the Sigma-algebra is that we can define a map from it to the real numbers. This map should measure the set in a sense of a generalised volume. Therefore, we just call this map a measure:
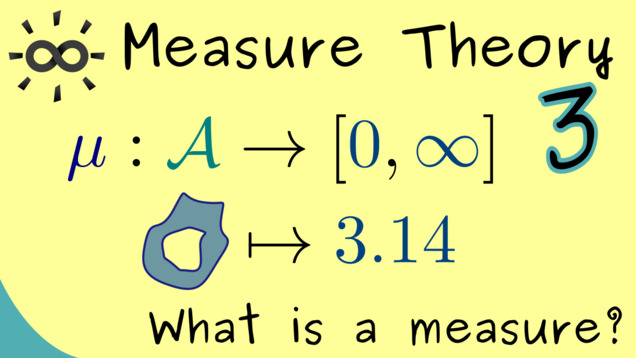
Content of the video:
00:00 Introduction
00:26 Measure - Definition
03:08 Empty set has measure zero
03:35 Additivity
05:59 Sigma-additivity
07:54 Summary
08:43 Measure space
09:18 Examples
09:40 Counting measure
12:30 Dirac measure
14:12 Lebesgue measure
Part 4 - Not everything is Lebesgue measurable
As an interlude I want to answer the question why we don’t just ignore Sigma-algebras and define the measure on the whole power set. This would mean that we can measure “volumes” of all possible subsets and wouldn’t that be a reasonable request? Yeah, it would be. However, then we would lose a lot of interesting measures, especially the most important one: The usual volume measure in $ \mathbb{R}^3 $. The next video proves that we cannot have this volume measure (and other ones) defined for the whole power set:
Part 5 - Measurable Maps
Now, we can go deeper into the theory. Keep in mind that someday we want to define a generalised integral with the help of such a measure. For that reason, we need to look at special maps that converse the structure of our $ \sigma $-algebra: measurable maps.
Part 6 - Lebesgue Integral
At this point, we can start by defining the so-called Lebesgue integral. It is the modern integral notion that even works in this abstract framework. However, let’s start with simple functions: step functions!
Part 7 - Monotone Convergence Theorem (and more)
Wow, now we know what $ \int f d \mu $ means. It is a number that really represents the orientated volume for the graph of the function. Everything seems easier to define than what you might know from the Riemann integral. However, the real advantages of the Lebesgue integral come into play when we look at the convergence theorems:
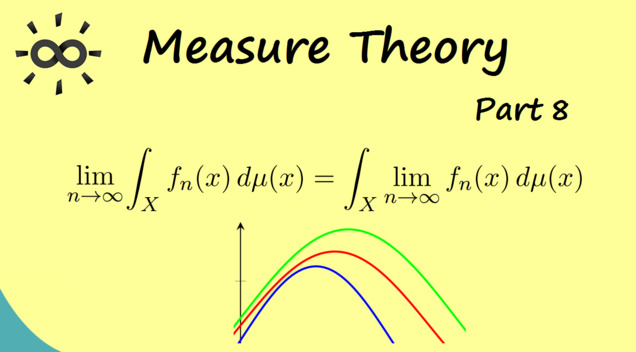
Part 8 - Monotone Convergence Theorem (Proof and Application)
Also a proof would be nice:
Part 9 - Fatou’s Lemma
Related to the convergence theorems is a very general fact, now often just called Fatou’s Lemma:
Part 10 - Lebesgue’s Dominated Convergence Theorem
At this point, you are so familiar with the Lebesgue integral that the next theorem might not surprise you so much. However, it is a property the simple one-dimensional Riemann integral lacks, but it has many applications. There, Lebesgue’s dominated convergence theorem is my favourite theorem in the integration theory:
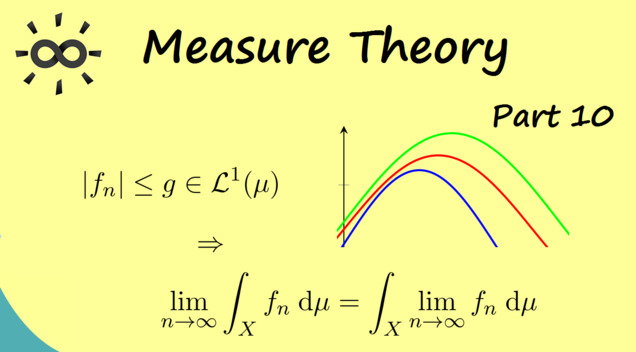
Part 11 - Proof of Lebesgue’s Dominated Convergence Theorem
Of course, you want to see a proof of this beautiful theorem, so here we go:
Part 12 - Carathéodory’s Extension Theorem
I hope that you now have a good overview about measures and the Lebesgue integral. We now go one step back and look at the foundations again. Especially, we are interested how we can construct some particular measures like the normal Lebesgue measure, for example. Therefore, let’s first talk about Carathéodory’s extension theorem:
Part 13 - Lebesgue-Stieltjes Measures
With the help of this theorem, we are able to construct a lot of different measures. One special class are so-called Lebesgue-Stieltjes measures:
Part 14 - Radon-Nikodym theorem and Lebesgue’s decomposition theorem
We reached a point where we talk about deeper and special results in Measure Theory. However, both theorem that now follow have so many applications that you could come across them everywhere. They are called the Radon-Nikodym theorem and Lebesgue’s decomposition theorem:
Part 15 - Image measure and substitution rule
For the Riemann integral, you know how to apply a substitution to solve some integrals. Of course, this also works for the Lebesgue integral in $\mathbb{R}$ in the same way. More interesting is the fact that you can generalise this rule to all other measurable spaces. There we need to define the image measure to get a similar substitution rule:
Part 16 - Proof of the substitution rule for measure spaces
Again, I should show you a proof of this:
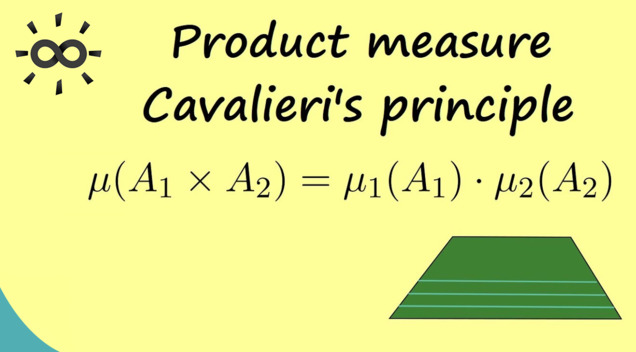
Part 17 - Product measure and Cavalieri’s principle
Now you know how to get new measures when you have a measurable map. It works similar when you want to define a new measure for the cartesian product. This one is called the product measure. With the help of this, we can simply higher-dimensional integrals. This is known as Cavalieri’s principle:
Part 18 - Cavalieri’s principle - An example
Of course, an example would be helpful to understand the whole procedure:
Part 19 - Fubini’s Theorem
We came far into the topic of measure theory and integration theory. I want to close the last topic but presenting one of the most famous theorems in this context: Fubini’s Theorem. You might already heard it and even applied it but I want to show you the general context such that you know what the correct assumptions are. I also show you an example 🙂
Part 20 - Outer measures - Part 1
The next videos are more about the foundations of measure theory. Especially we first talk about outer measures in the next three videos:
Part 21 - Outer measures - Part 2: Examples
Let’s discuss some examples.
Part 22 - Outer measures - Part 3: Proof
Now, let’s prove the statements.
Part 23 - Proof of Carathéodory’s Extension Theorem
In the final video of the series, we put everything together to prove Carathéodory’s Extension Theorem. It turns that we already have almost everything we need from the discussion of the outer measures. The only gap we have to close is the step from a premeasure to an outer measure. Let’s do that.
This ends the series about constructing measures and general Lebesgue integral. It’s something we can use in the Probability Theory course to construct general probability measures. In the future, we will also discuss more properties of measures in an additional course.
Summary of the course Measure Theory
- You can download the whole PDF here and the whole dark PDF.
- You can download the whole printable PDF here.