-
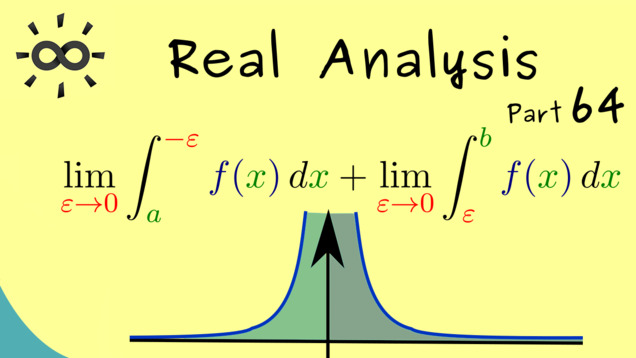
Title: Cauchy Principal Value
-
Series: Real Analysis
-
Chapter: Riemann Integral
-
YouTube-Title: Real Analysis 64 | Cauchy Principal Value
-
Bright video: https://youtu.be/0SP2b0nFpwI
-
Dark video: https://youtu.be/wlg0n5_t_6M
-
Ad-free video: Watch Vimeo video
-
Forum: Ask a question in Mattermost
-
Quiz: Test your knowledge
-
Dark-PDF: Download PDF version of the dark video
-
Print-PDF: Download printable PDF version
-
Exercise Download PDF sheets
-
Thumbnail (bright): Download PNG
-
Thumbnail (dark): Download PNG
-
Subtitle on GitHub: ra64_sub_eng.srt missing
-
Download bright video: Link on Vimeo
-
Download dark video: Link on Vimeo
-
Timestamps (n/a)
-
Subtitle in English (n/a)
-
Quiz Content
Q1: Let $f: [0,2]\setminus{1} \rightarrow [0,1]$ be an unbounded function. What is the correct definition of the improper Riemann integral $$ \int_0^2 f(x), dx$$
A1: $$ \lim_{\varepsilon \searrow 0 } \int_0^{ 1 - \varepsilon} f(x), dx + \lim_{\varepsilon \searrow 0 } \int_{ 1 + \varepsilon}^{2} f(x), dx $$
A2: $$ \lim_{\varepsilon \searrow 0 } \int_0^{ 2 - \varepsilon} f(x), dx + \lim_{\varepsilon \searrow 0 } \int_{ 1 + \varepsilon}^{2} f(x), dx $$
A3: $$ \lim_{\varepsilon \searrow 0 } \int_0^{ 2 - \varepsilon} f(x), dx + \lim_{\varepsilon \searrow 0 } \int_{ 2 + \varepsilon}^{2} f(x), dx $$
A4: $$ \lim_{\varepsilon \searrow 0 } \int_0^{ 2 - \varepsilon} f(x), dx + \lim_{\varepsilon \searrow 0 } \int_{\varepsilon}^{2} f(x), dx $$
Q2: $f: [-1,1] \setminus {0} \rightarrow [0,1]$ be given by $ f(x) = \frac{1}{x}$. Does the improper Riemann integral exist?
A1: Yes!
A2: No!
Q3: $f: [-1,1] \setminus {0} \rightarrow [0,1]$ be given by $ f(x) = \frac{1}{x}$. Does the Cauchy principal value exist?
A1: Yes!
A2: No!
Q4: $f: [-1,1] \setminus {0} \rightarrow [0,1]$ be given by $ f(x) = \frac{1}{x^3}$. What is the Cauchy principal value $$\mathrm{p.v.} \int_{-1}^1 \frac{1}{x^3} , dx $$ of this function?
A1: $0$
A2: $1$
A3: $\frac{1}{2}$
A4: $\frac{1}{3}$
A5: $3$
-
Last update: 2025-01