Information about Multivariable Calculus - Part 9

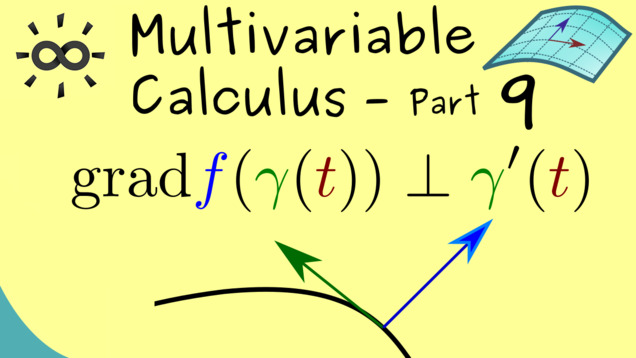
Title: Geometric Picture for the Gradient

Series: Multivariable Calculus

YouTube-Title: Multivariable Calculus - Part 9 - Geometric Picture for the Gradient

Bright video: https://youtu.be/P0EyEdEcS3c

Dark video: https://youtu.be/R4gbrX6sZuU

Quiz: Test your knowledge

PDF: Download PDF version of the bright video

Print-PDF: Download printable PDF version

Thumbnail (bright): Download PNG

Back to overview page