Q1: Let $\mathcal{B} = (\mathbf{u}, \mathbf{v})$ be a basis of a subspace $U\subseteq \mathbb{R}^n$. Which family is also a basis for $U$?

A1: $(3\mathbf{u}, 4\mathbf{v})$

A2: $(\mathbf{u}, \mathbf{u})$

A3: $(\mathbf{u}, -\mathbf{u})$

A4: $(2\mathbf{v}, -\mathbf{v})$

A5: $(2\mathbf{v}, 4\mathbf{v})$

Q2: What is the formulation of Steinitz Exchange Lemma as we have learnt in the video.

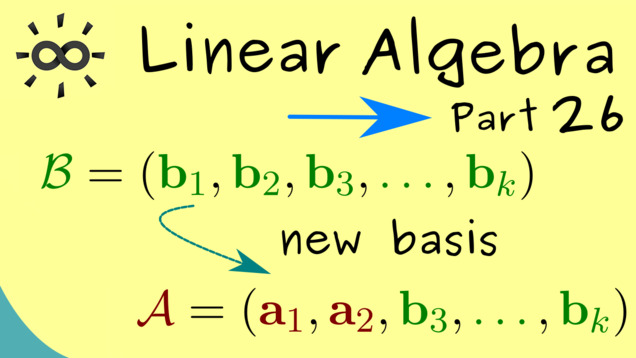
A1: Let $\mathcal{B}$ be a basis of a subspace $U$ with $k$ vectors and $\mathcal{A}$ be a family of $\ell$ linearly independent vectors in $U$. Then one can add $k - \ell$ vectors from $B$ to $\mathcal{A}$ to get a new basis of $U$.

A2: Let $\mathcal{B}$ be a basis of a subspace $U$ with $k$ vectors and $\mathcal{A}$ be a family of $\ell$ linearly independent vectors in $U$. Then one can remove $k$ vectors from $B$ to $\mathcal{A}$ to get a new basis of $U$.

A3: Let $\mathcal{B}$ be a basis of a subspace $U$ with $k$ vectors and $\mathcal{A}$ be a family of $\ell$ linearly independent vectors in $U$. Then $\mathcal{A} \cup \mathcal{B}$ is new basis of $U$.