-
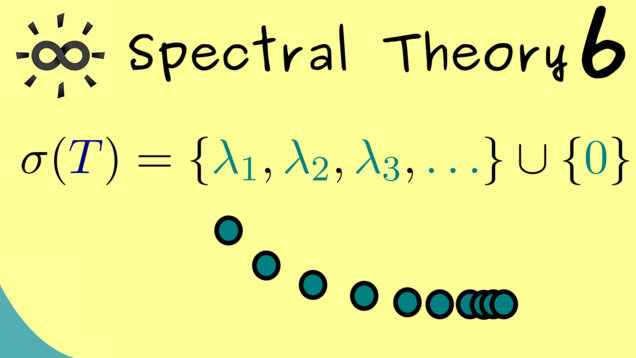
Title: Spectrum of Compact Operators
-
Series: Functional Analysis
-
YouTube-Title: Functional Analysis 33 | Spectrum of Compact Operators
-
Bright video: https://youtu.be/vAO57QjSCCo
-
Dark video: https://youtu.be/PmJIYVT4TGI
-
Ad-free video: Watch Vimeo video
-
Quiz: Test your knowledge
-
Dark-PDF: Download PDF version of the dark video
-
Print-PDF: Download printable PDF version
-
Thumbnail (bright): Download PNG
-
Thumbnail (dark): Download PNG
-
Subtitle on GitHub: fa33_sub_eng.srt missing
-
Timestamps (n/a)
-
Subtitle in English (n/a)
-
Quiz Content
Q1: Let $X,Y$ be two normed spaces and $T: X \rightarrow Y$ a bounded linear operator. Which is the correct condition for $T$ being a compact operator?
A1: $\overline{T[ B_1(0) ]}$ is compact.
A2: $ T[ B_1(0) ]$ is closed.
A3: $ T[ B_1(0) ]$ is compact.
A4: $ T[ B_1(0) ]$ is open.
A5: $ T[ B_1(0) ]$ is bounded.
A6: $\overline{T[ B_1(0) ]}$ is finite.
Q2: Let $X$ be a complex Banach space and $T: X \rightarrow X$ a compact operator. Which claim is, in general, not correct?
A1: $\sigma(T)$ is always a finite set.
A2: $\sigma(T)$ is always a countable set.
A3: $\sigma(T) \setminus { 0 }$ is always a countable set.
A4: $\sigma(T) \setminus { 0 }$ could be the empty set.
Q3: Let $X$ be an infinite-dimensional complex Banach space and $T: X \rightarrow X$ a compact operator. Which claim is, in general, not correct?
A1: $\sigma(T) \cap { 0 }$ is the empty set.
A2: $\sigma(T) \cap { 0 }$ is a finite set.
A3: $\sigma(T) \setminus { 0 }$ is a countable set.
A4: $\sigma(T)$ contains at least one element.
-
Last update: 2024-10