-
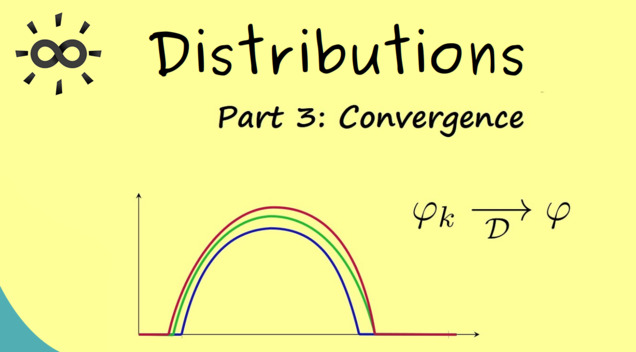
Title: Convergence of Test Functions
-
Series: Distributions
-
YouTube-Title: Distributions 3 | Convergence of Test Functions
-
Bright video: https://youtu.be/yefkxL-yYjo
-
Dark video: https://youtu.be/o4E4MKPKAKg
-
Ad-free video: Watch Vimeo video
-
Original video for YT-Members (bright): https://youtu.be/HopDSb2ulc4
-
Original video for YT-Members (dark): https://youtu.be/rhHa6zwrP-I
-
Quiz: Test your knowledge
-
Dark-PDF: Download PDF version of the dark video
-
Print-PDF: Download printable PDF version
-
Thumbnail (bright): Download PNG
-
Thumbnail (dark): Download PNG
-
Subtitle on GitHub: dt03_sub_eng.srt missing
-
Timestamps (n/a)
-
Subtitle in English (n/a)
-
Quiz Content
Q1: Let us consider the set of test functions $\mathcal{D}(\mathbb{R}^n) = C_c^\infty( \mathbb{R}^n)$. What do we mean when we write $$ \varphi_k \xrightarrow[\mathcal{D}]{k \rightarrow \infty} \varphi $$
A1: The sequence of functions is uniformly convergent.
A2: There is bounded set such that all supports of $\varphi_k$ lie inside it and the sequence of functions is uniformly convergent.
A3: There is bounded set such that all supports of $\varphi_k$ lie inside it and $$D^\alpha(\varphi_k) \rightarrow D^\alpha(\varphi)$$ uniformly for all multi-indices.
Q2: Does the sequence $(\varphi_k)$ with $\varphi_k = 0$ converge in the space $\mathcal{D}(\mathbb{R}^n)$?
A1: Yes.
A2: No.
Q3: Consider the function $\varphi_k \in \mathcal{D}(\mathbb{R}^n)$ given by $$\varphi_k(x) = \begin{cases} 0 &,~ | x | \geq 1 \ \frac{1}{k} \exp\left( - \frac{1}{1 - |x |^2 } \right) &,~ | x | < 1 \end{cases}$$ Which claim is true?
A1: $$ \varphi_k \xrightarrow[\mathcal{D}]{k \rightarrow \infty} 0 $$
A2: $$ \varphi_k \xrightarrow[\mathcal{D}]{k \rightarrow \infty} \varphi_1 $$
A3: $$ \varphi_k \xrightarrow[\mathcal{D}]{k \rightarrow \infty} 1 $$
A4: $$ \varphi_k \xrightarrow[\mathcal{D}]{k \rightarrow \infty} \varphi_2 $$
-
Last update: 2024-11