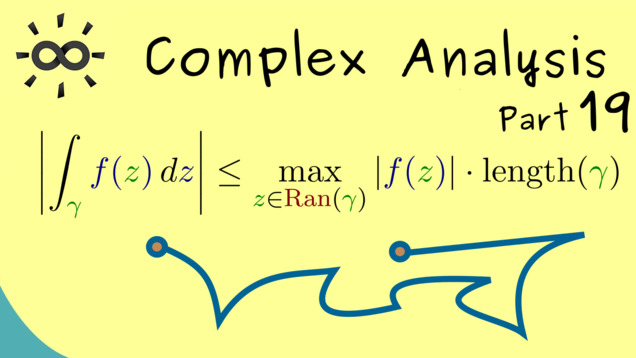
-
Title: Properties of the Complex Contour Integral
-
Series: Complex Analysis
-
YouTube-Title: Complex Analysis 19 | Properties of the Complex Contour Integral
-
Bright video: https://youtu.be/yLtWKLroQL0
-
Dark video: https://youtu.be/b_9V_WhM1uM
-
Ad-free video: Watch Vimeo video
-
Original video for YT-Members (bright): https://youtu.be/fcHxCHI-01s
-
Original video for YT-Members (dark): https://youtu.be/NaFjC36HeRk
-
Forum: Ask a question in Mattermost
-
Quiz: Test your knowledge
-
Dark-PDF: Download PDF version of the dark video
-
Print-PDF: Download printable PDF version
-
Thumbnail (bright): Download PNG
-
Thumbnail (dark): Download PNG
-
Subtitle on GitHub: ca19_sub_eng.srt missing
-
Download bright video: Link on Vimeo
-
Download dark video: Link on Vimeo
-
Timestamps (n/a)
-
Subtitle in English (n/a)
-
Quiz Content
Q1: Let $\gamma: [0,1] \rightarrow \mathbb{C}$ be piecewise continuously differentiable. What is not correct?
A1: $\gamma$ is continuous.
A2: There are $a_i \in [0,1]$ such that $\gamma|{[a_i,a{i+1}]}$ is continuous.
A3: There are $a_i \in [0,1]$ such that $\gamma|{[a_i,a{i+1}]}$ is differentiable.
A4: There are $a_i \in [0,1]$ such that $\gamma|{[a_i,a{i+1}]}$ is continuously differentiable.
A5: There are $a_i \in [0,1]$ such that $\gamma|{[a_i,a{i+1}]}$ is two-times differentiable.
Q2: Let $\gamma: [0,2 \pi] \rightarrow \mathbb{C}$ be given by $\gamma(t) = e^{it}$ and $f(z) = \frac{1}{z}$. What is $ \oint_\gamma f(z) , dz $?
A1: $0$
A2: $1$
A3: $2 \pi i$
A4: $i$
Q3: Let $\gamma: [a,b] \rightarrow \mathbb{C}$ be a piecewise continuously differentiable. What do we need to say that $\gamma$ is closed?
A1: $\gamma(a) = 0$
A2: $\gamma(a) = \gamma(b)$
A3: $\gamma(a) = \gamma(b) = 0$
A4: $\gamma(a) = 1$
Q4: Let $\gamma: [a,b] \rightarrow \mathbb{C}$ be a piecewise continuously differentiable and $\gamma^-$ the curve with reversed orientation. What is correct?
A1: $ \int_\gamma f(z) , dz = 0$
A2: $ \int_\gamma f(z) , dz = \int_{\gamma^-} f(z) , dz$
A3: $ \int_\gamma f(z) , dz = -\int_{\gamma^-} f(z) , dz$
A4: $ \int_\gamma f(z) , dz = 2 \int_{\gamma^-} f(z) , dz$
-
Last update: 2024-10
